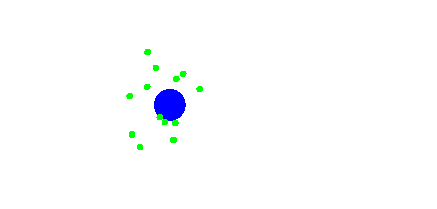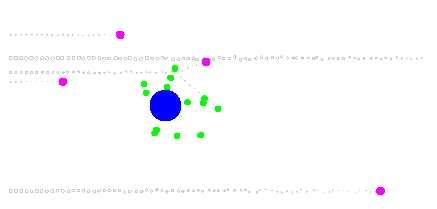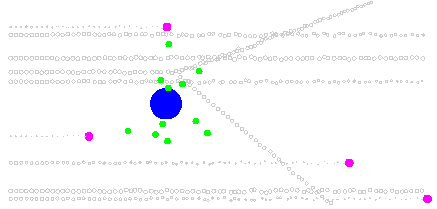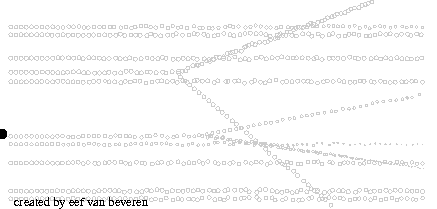
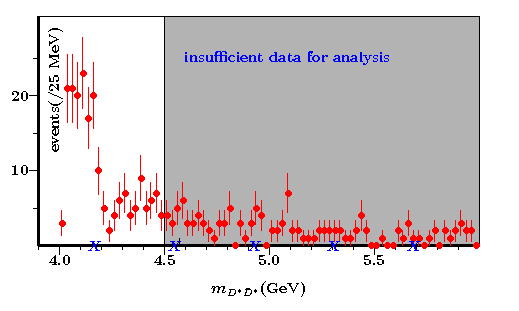
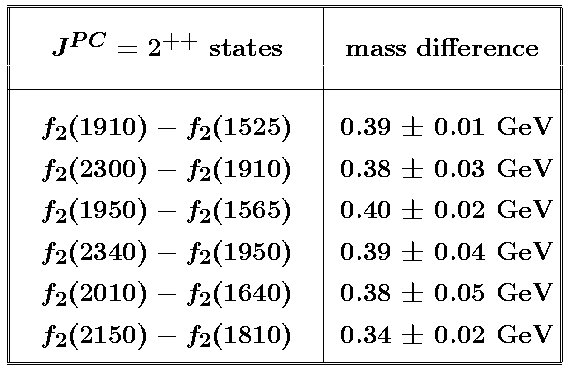
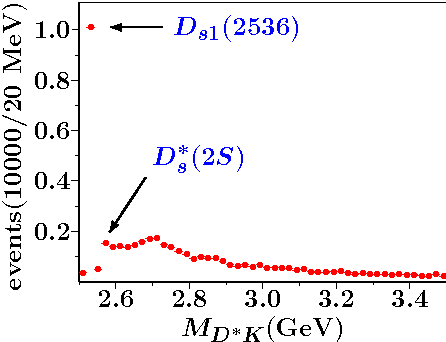
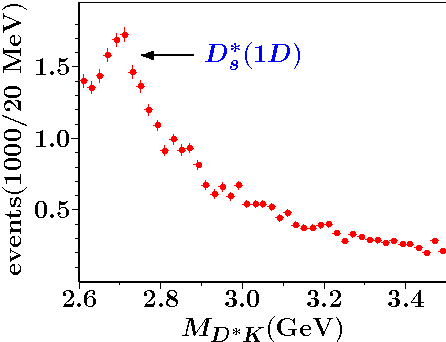
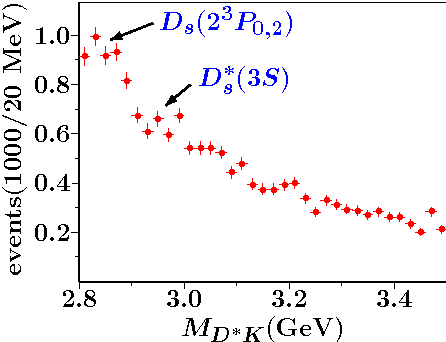
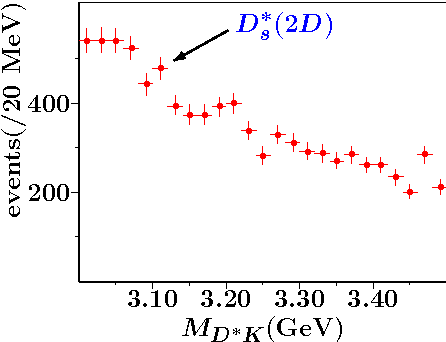
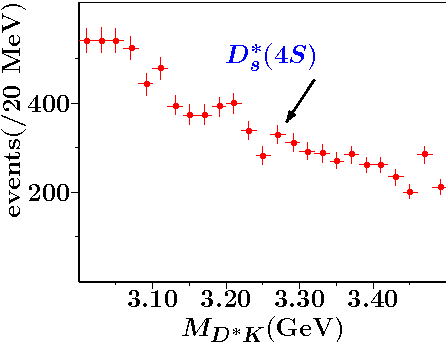
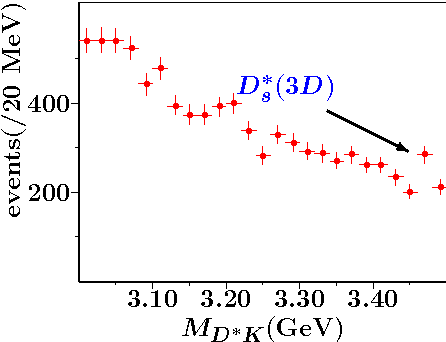
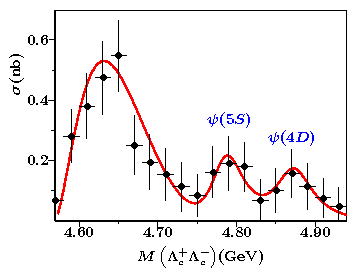
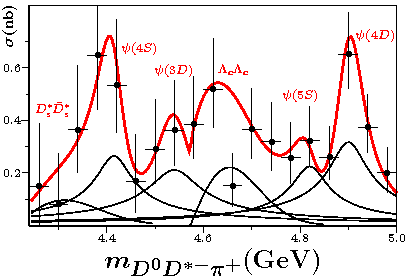
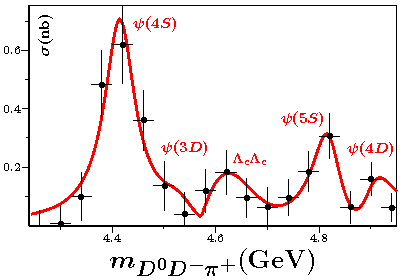
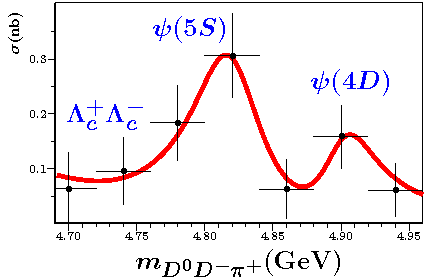
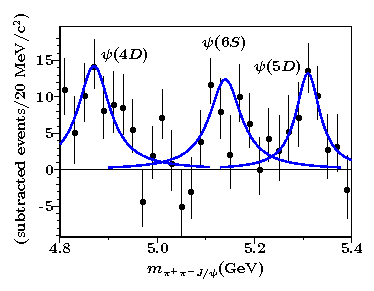
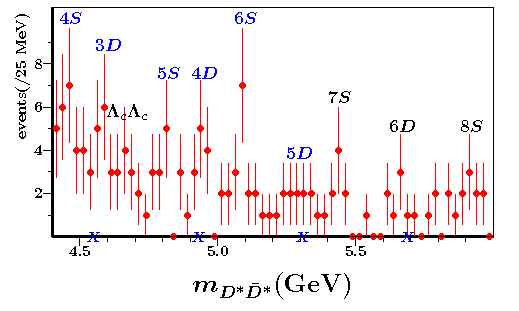
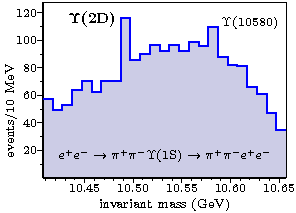
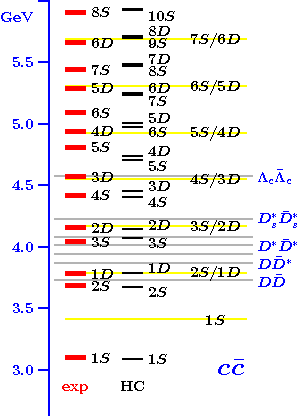
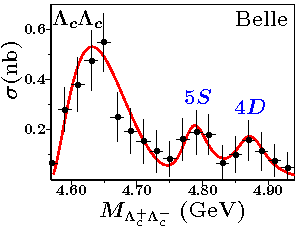
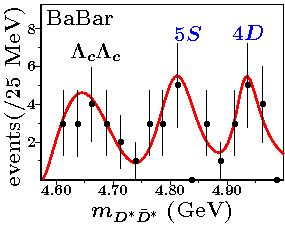
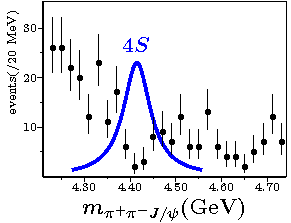
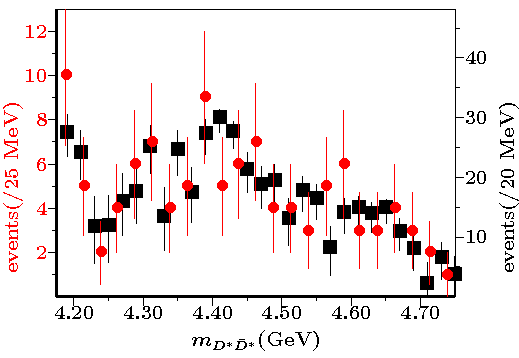
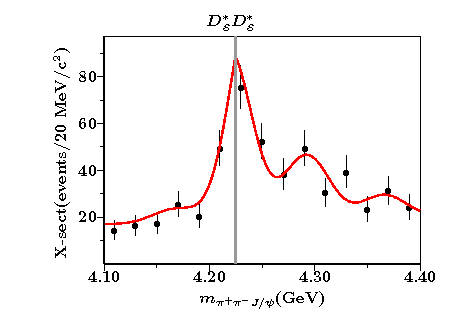
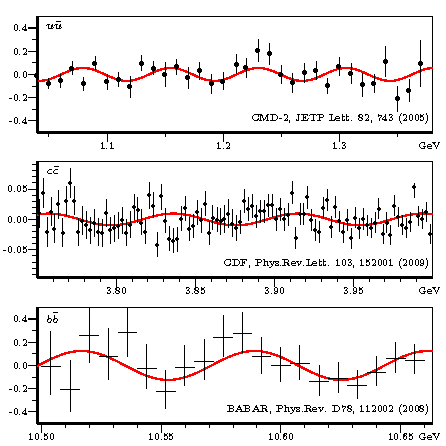
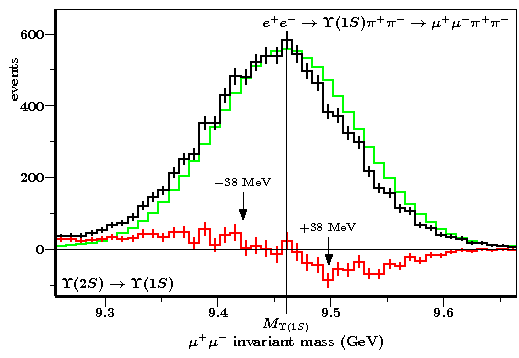
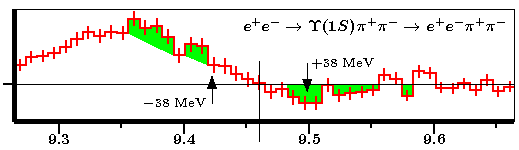
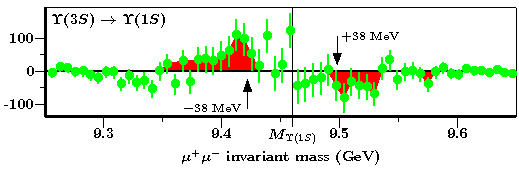
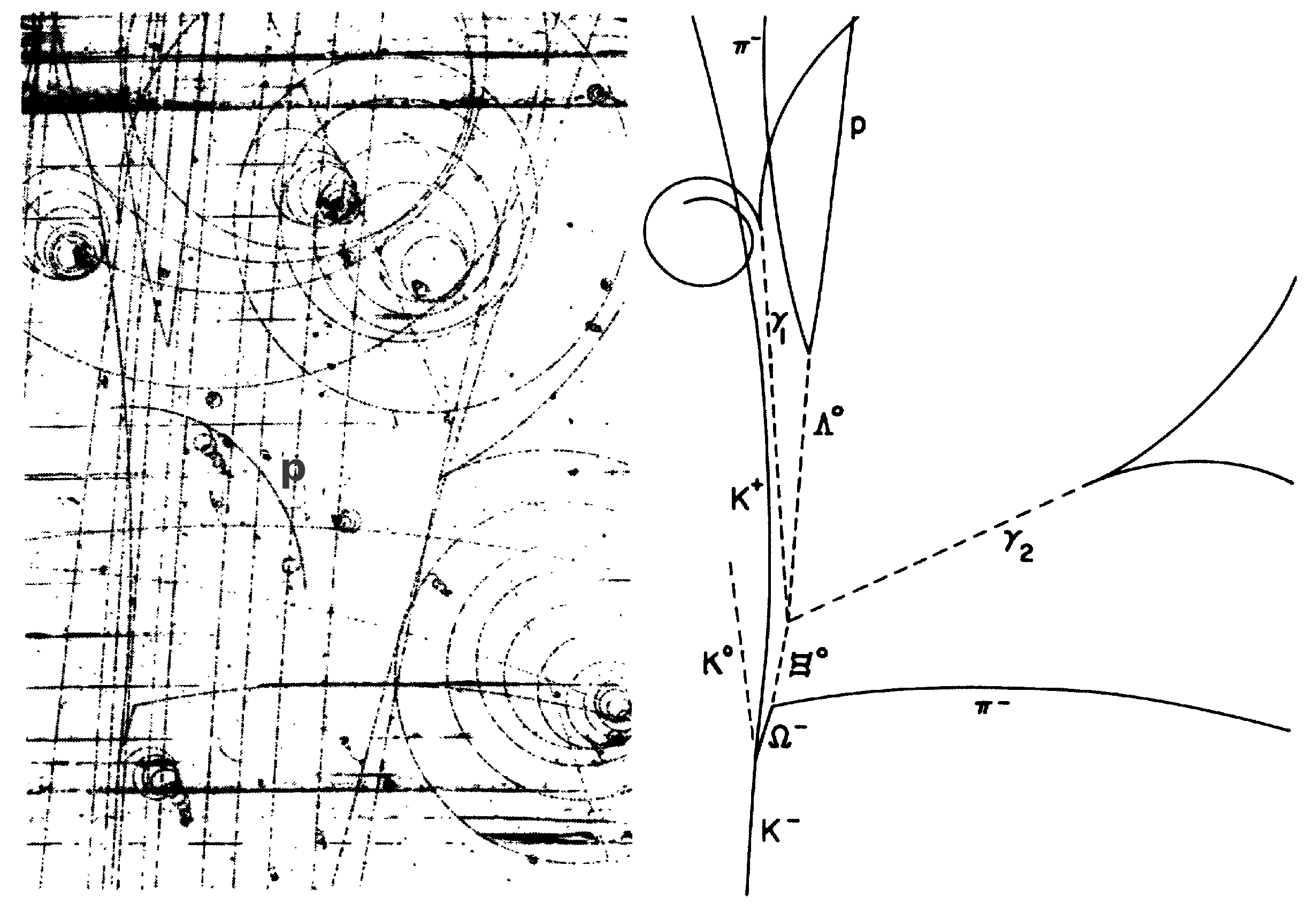
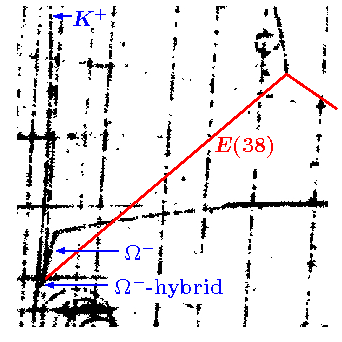
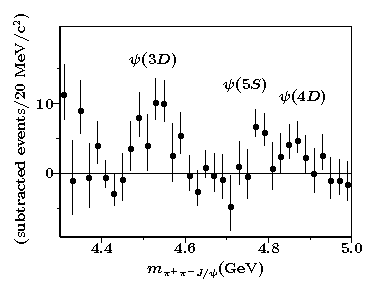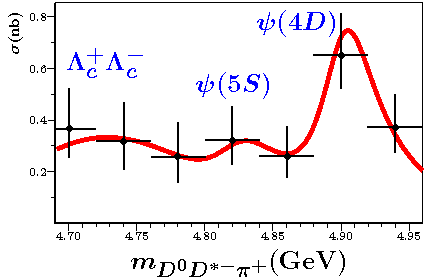The higgs bosons of strong interactions.

Very recently, I found extremely clear evidence
in photon-photon (γγ) data,
more than 13σ (5σ is considered enough),
for the existence of a very light boson in existing data:

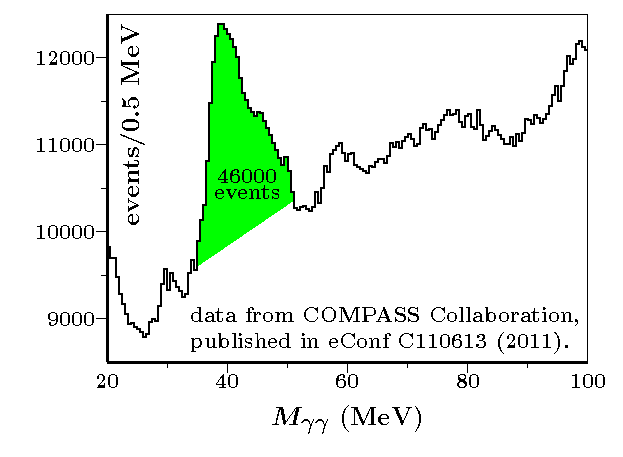
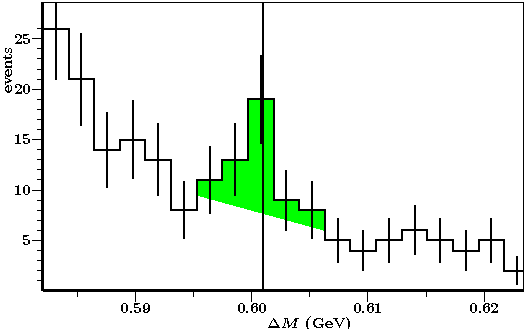
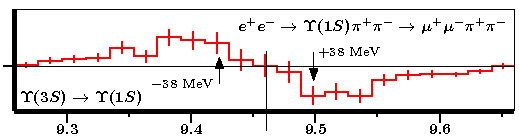
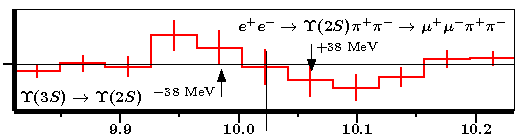
in pπ-→pπ-η(958) by COMPASS (CERN) (pdf version)


and, moreover, a few more indications of less statistical significance:


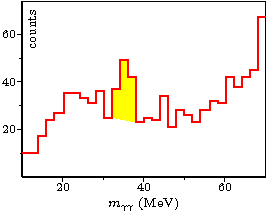
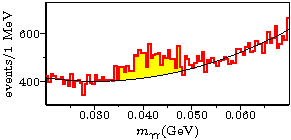
  in pγ→pγγ by CB-ELSA (Jülich)  
  in pp→ppω/φ by COMPASS (CERN)  


Possible clean fuel, since it leave no residues,
for nuclear-energy batteries in space ships?

In the video I describe where we were in 2011.


Published experimental results
hint at the existence of very light scalar bosons,
the higgs bosons of strong interactions.


Skip all introduction


The E(38)

The E(38) is a new nuclear particle that has not been discovered before
because of its very small interaction with normal matter.
However it has recently been identified by me in high-energy processes,
because at such energies also heavy fundamental particles, like the top quark, play their role.
I have published this result together with my collaborator George Rupp
from the Technical University of Lisbon.

I suspected the existence of such light fundamental particle for three decades
and found in the past already several miniscule signs of it in experimental data
which were obtained at large acellerator laboratories and published in scientific journals.
Recently, I discovered a clear sign of the E(38) in data obtained
by the COMPASS Collaboration at CERN.

Although little more is known by now than that it exists,
I suspect that it is a kind of glue bubble, a miniature soap bubble.

The E(38) might explain the discrepancy in the size of the proton radius
between muonic and electronic data, because the E(38) interacts stronger
with strange quarks than with the light quarks.

When there exists a stable form of the E(38), then it might even be a source of clean nuclear fuel,
because at desintegration it deposits all its heat and does not leave any deposit.
Such fuel could play a role in traveling through space
for the exploration of new continents at distant planets.

But, first its properties should be established in dedicated experiments.

Yet one more particle?

The reason for such question is the pollution of the word "particle".
Many times in the recent past, the word has been used while refering to resonances,
or excitations, of systems which consist of quarks.
Would one use the same terminology for excitations of the hydrogen atom,
than hundreds of hydrogen particles have been identified
since their discovery by Wollaston and Fraunhofer two centuries ago.

Initially one thought of hadrons as new particles.
However, we now know that they are built of something more fundamental,
quarks and gluons.
Hence, excitations of those systems should no longer be refered to as particles,
in order not to confuse them with the fundamental building blocks of nature.

At present the latter are the quarks and gluons, photons, neutrino's,
electrons and the heavy gauge bosons W and Z.

The E(38) is a new member of that class of particles.

Higgs-type particle?

As far as I could extract from experimental data,
it has the kind of properties that the hypothetical Higgs particles should have.
Hence, the E(38) could very well be the Higgs particle for strong interactions
which I predicted some thirthy years ago in a model for such particle
constructed by me and two Dutch scientists,
thereby unifying electromagnetism and strong interactions.



Particle detection
Go to bubble chamber photograph

A very unexpected result. Not at about 125 GeV, as in the recent Fermilab and CERN results, but a three-orders-of-magnitude lighter particle, in a domain where everything was supposed to be solved and a closed chapter in physics research.

A light scalar boson with a mass of 38 MeV and two replicas at twice and three times that mass is the most spectacular finding of the past decades in particle physics.

A mass of 38 MeV means that the light scalar higgs boson for strong interactions is about 25 times lighter than a proton or a neutron, whereas it is almost 75 times more heavy than an electron.

The lightest hadrons observed so far are the pions, with masses 135 MeV (neutral pion) and 140 MeV (charged pions), more than three times, almost four times, more heavy than the light scalar higgs boson for strong interactions. Moreover, the pions consist of quarks, whereas we suspect that the light scalar higgs boson for strong interactions is empty, only made of the glue which holds the quarks together in hadrons.

Most of my colleagues are skeptical towards my finding. They argue that the lightest ball of glue which is predicted by lattice QCD is at least some 500 times more heavy. But, lattice QCD also predicts that the excited states of hadrons come closer together in energy, when one goes higher up in the quark spectrum, whereas it is observed that the mass differences in the meson spectrum for higher excited states are constant and equal to 380 MeV. This had been predicted by me and my collaborators already three decades ago. But when, thirty years later, it was finally observed in published experimental results of the BABAR collaboration, who did their experiments at the SLAC collider (in Stanford CA, USA), the experiment was stopped and their results not further inspected. The signals which I observed in the published BABAR results are feeble and therefor not recognised by my colleagues. Nevertheless, from the fact that no better signals have been produced by experimental collaborations, one cannot conclude much more than that the small signals we are provided with do not point towards the lattice QCD predictions.

Other colleagues argue that such light particle should have been observed in several experiments. However, when I insist on giving me then the precise processes through which it could have been discovered before, their arguments fall short and no viable suggestion comes up. Actually, I only see two possible processes which could eventually be studied by experimentalists. One is two-photon decay, but it will be a very rare event, leading to a small and narrow signal. Hence, quite some experimental precision is needed for it. The other is through missing mass, which is exactly what I did in the beautonium sector, nevertheless leading however to very feeble signals.

Three decades ago I found that light-quark-pair creation had to be related to a quantum of about 30-40 MeV. I waited many years for experimental data that could confirm its existence. A first hint came from the oscillations in annihilation data of a BABAR experiment. Subsequently, I found similar results in other data. Those results, which are neither predicted by lattice QCD, nor by the Standard Model, show that experimental cross sections for meson-pair production oscillate with a rythme which agrees nicely with the interference of the light scalar higgs boson for strong interactions and the 380 MeV level spacings of the meson spectra. However, only after observing the signature of a 38 MeV scalar boson in also beautonium transitions, I decided to challenge experimentalists by claiming its possible existence since we have by now two pieces of theoretical evidence and five pieces of experimental evidence which is more than enough to prove its existence.

Particle supersymmetry and strings are very interesting developments in mathematics and physics. Whether this branch has anything to do with nature, still has to be seen. The scalar boson which I describe here, is not a supersymmetric particle, but is the quantum which is responsible for quark-pair creation. As such it could have lived exclusively in the deep interior of hadrons. The surprise of my observation is that it seems to be capable to exist on its own, exterior to the protective surrounding of hadronic matter.

If people get convinced it exists, then still a lot of work must be done to obtain a better proof. One thing is indicating its possible existence by pointing out a number of feeble signals in a variety of experimental results, yet another thing is setting up an experiment which collects sufficient data to show the existence of those light scalar bosons beyond any doubt. A lot of people will be involved in such a project.

The higgs scalar boson for strong interactions, which is described in the present video, is not the same as the Standard-Model higgs boson.

The Standard-Model higgs boson is about the weak and electromagnetic forces for which the Standard Model has been developed. From Fermi's theory for weak interactions it could be predicted that those interactions are mediated by the exchange of heavy bosons, the W+ and W-. As a consequence, also a neutral heavy boson had to exist, the Z boson. All three heavy bosons have been found in experiment. But the theory for weak interactions could only be completed by involving the electromagnetic forces as well. That construction, the Standard Model, needs an additional heavy boson, the Standard-Model higgs. However, there is not a clue about its mass in the Standard Model.

The higgs scalar boson for strong interactions is something similar for strong interactions. Its mass could be predicted from experimental results, as described in the present video. Nevertheless, it lasted three decades before direct indications for its existence could be observed from experimental results. That is the issue of the video and the related publication. Not the Standard-Model higgs.

About the existence of the higgs scalar boson for strong interactions, which is the subject of the present video, can be no doubt. The various signals from experimental results leave very little room for different explanations. It has also been predicted in theoretical works.

Nature has many secrets. Sometimes we seem to understand and be capable to do predictions on the outcome of experiments. I could not perform such experiments myself. So, I had to wait many years before results came available which show the existence of light scalar higgses. At Fermilab and CERN one is trying to demonstrate the existence of heavy higgses which are predicted by the Standard Model. Without faith all of us would have given up long ago

I believe in down-to-earth physics which has a close connection to what can be observed in experiment. Hence, I consider mathematically consistent frameworks which extrapolate too far beyond where experiment can falsify its predictions, as a lot of fun, but not to be taken too seriously. There exist many examples, the Standard Model for particle physics is just one of them. Deep in my heart I pray that the Creator had a bit more imagination, way beyond our horizon, when she cooked up nature.

Our perception of the Universe in which we live has evolved rapidly in the twentieth century. Was it common knowledge at the onset of that century that our Earth is just a modest size planet of the solar system, now we are aware that the center of that system, our Sun, is just a very modest star which belongs to a modest size galaxy in a Universe filled with galaxies. It dazzles us if we just try to imagine how we may explore that new world, opened to us, but still far beyond our reach.

One has discovered other solar systems with planets similar to ours, just as we had imagined it. Whether there would be life on them has become one of the most intriguing puzzles for the future and has triggered speculations that one day Earth might be discovered and explored by creatures far more developed than us.

In past centuries, people traveled to the unknown in order to conquer the world. We live now everywhere on our planet. Clearly, the next step is traveling to the unknown world of new planets. Several projects are underway to ensure that future generations will be capable to conquer the Universe, or at least be sufficiently educated to meet with visitors from space. Most of the world population is well aware of the gigantic tasks awaiting us in the times to come. Furthermore, planning and education give globalization nowadays the highest priority, whereas internet provides for the spreading of knowledge and know-how.

Many persons have contributed to the progress of knowledge. Some even dedicated their entire life to explore an unknown territory of the mysteries behind natural phenomena, this way providing mankind with smaller or larger steps forward in dominating the forces of nature. Written history of all cultures tells us about man's curiosity to uncover answers to their mysteries. Now man is looking outward from Earth into far away distances and dreams about the necessary means which would allow him to become the master of the Universe. But, there is yet another reason for his anxiety to get into outer space, namely that he does not even know for how long our planet will hold on to harbour us. The future of mankind depends on exploring the Universe.

Each new discovery, how insignificant it may seem today, puts one more piece in a gigantic puzzle. But, are we capable to perform the unavoidable tasks? Can we combine serious thoughts about the survival of mankind with unlimited enjoying the larger and smaller pleasures of life? For that we have to learn that discovering things is one of the bigger pleasures of life. It is reassuring that such attitude is nowadays being stimulated in education at home as well as at schools. Furthermore, it is a fortunate development that results at present are being exposed and discussed in public media and social communication networks, not just in small circles of so-called experts.

Our Universe is so much larger than our Earth, our solar system, or even our Milky Way galaxy that it seems natural that man started by making an inventory of what can be observed, planets, stars and galaxies, before he seeks to understand how to conquer it. Mapping the stars finds its roots in ancient history when it was related to supernatural powers and religion. Hence, without even understanding the precise goal of their investigations, those women and men somehow convinced themselves of the importance of their scientific activity. Cosmology is nowadays a vast domain of research to which many women and men contribute and in which every single day new discoveries are made. One has gained knowledge on star aging and has observed objects which are probably neutron stars and black holes. But, however important, inventory alone is not enough to reach the stars. We will also need means of transportation.

At present we cannot even reach the planets of our solar system. Yes, man has landed on the Moon and returned safely home from it. But, we have to multiply the distance Earth-Moon by a factor of one billion or more to reach te nearest planets outside our solar system. Consequently, with present techniques we would have to travel several billions of weeks, which is hundreds of millions of years, before we finally could stretch our legs on a new planet. Clearly, not yet suitable for mankind to populate even the nearest planets, let it be to conquer the Universe. But, it took man only a few generations to reduce trips of several weeks by road and over sea, to trips of a few hours by air.
We might thus just need some more knowledge on how things work.

We know of the existence of cosmological particles which transport themselves at very high velocities approaching the light velocity which is the limit of speed as set by Albert Einstein. But, even if it were possible to dispose over spacecrafts at such speeds, it would escape human capability to maneuver it. Moreover, we would need to be able to control all forces which dominate the fabric of nature in order to transport safely the humans inside such spaceship. For that we will have to make carefully an inventory of all existing forces. Hence, one of the main challenges for the future is to gain complete knowledge on the driving forces underlying the constellation of our Universe. Contrary to what the dimensions of the Universe suggest, those forces seem to be contained in the very small, in the world of subatomic systems.

The discovery of phenomena related to electricity and magnetism in previous centuries has led to much of the comforts of our present society, internet being one of the latest developments. But, we should not easily forget the tremendous efforts of all those women and men who, centuries ago, pieced it together. Those people had no idea to where it would lead. They were merely driven by a conviction which made them feel that each little discovery was needed for something great in the future. Not for themselves but for later generations, as the pace of discoveries is slow, in particular when only a few seem to comprehend their importance, or when results are even rejected and further research efforts forbidden out of fear that the world would slip out of the control of some Happy Few.

For example, a Greek mathematician, Eratosthenes of Cyrene, who was also a poet, athlete, geographer, astronomer and music theorist, was the first person to calculate with great precision the circumference of the earth in the third century BC, thereby assuming that our planet was a sphere. He may also have accurately calculated the distance from the earth to the sun and invented the leap day. Furthermore, he created a map of the world based on the available geographical knowledge of the era. He invented a system of latitude and longitude. Nevertheless, almost two thousand years later Giordano Bruno, an Italian Dominican friar, philosopher, mathematician and astronomer, was burned at the stake by civil authorities in 1600 after the Roman Inquisition found him guilty of cosmological theories that went beyond the Copernican model in proposing that the Sun was essentially a star, and moreover, that the universe contained an infinite number of inhabited worlds populated by other intelligent beings.

Gravity is the force which holds together the Universe. All objects in the Universe attract each other. The Earth and the Sun attract each other by a gravitational force. For that reason the Earth orbits around the Sun. Stars orbit around the center of their galaxies and galaxies also attract each other to remain in stable orbits. Although it seems that all stars are always in the same places, actually they move. But, it would need millions of years to notice that movement. Not because they move slowly, but because distances are so extremely large.

Gravity is not yet fully understood. For example, gravitational waves have not been discovered, whereas, traveling in time has inspired to the boldest imagination. The internet is inundated with tales of time travel. In science fiction, space and time warps are used for rapid journeys around the galaxy, or for travel through time. If time travel is really possible then man will discover it one day. It has happened before that yesterday's science fiction became today's reality. However, as yet we do not have the slightest clue. Ideas may be inspiring. But, they must be put to test in experiments which usually involve a lot of engineering. So, anybody who wants to accompany this line of research is welcome. Future generations will be grateful for even the smallest piece of the puzzle.

Electricity is the force which holds together atoms and molecules. The atomic nucleus attracts the electrons by the force of electricity. That is why electrons orbit the nucleus. Several atoms can share their electrons in order to form molecules. A molecule is thus a collection of atoms which form a stable constellation by means of the electric force. Each material is made out of completely identical molecules which hang together by also electric forces. For example, water is made out of water molecules which each consist of one oxygen atom and two hydrogen atoms. Water vapor consists of water molecules which can freely move through space at arbitrary distances. Liquid water consists of water molecules which can freely move through space but are all the time in close contact. Ice consists of water molecules which cannot move freely, but stick closely together at fixed places. Some materials consist of monoatomic molecules, for example metals, other of more complex molecules. Plastics have molecules which contain tens of thousands of atoms.

The force which is responsible for the properties of all materials, is electricity. Magnetism is a form of electricity. If magnetic monopoles would exist then magnetism would be a force on its own. Although magnetic monopoles have never been found, one usually uses the term electromagnetism to designate the force of electricity. Light is understood to be an electromagnetic wave.

The study of subatomic systems has made us aware of the existence of a world which was beyond the imagination of man one century ago. It all started in the beginning of the twentieth century with the discoveries of the structure of atoms. The picture emerged of a heavy nucleus orbited by a cloud of very light electrons. The electrons contribute with less then half per thousand to the total mass of the atom. The remaining almost one hundred percent of the mass of an atom is concentrated in the very small nucleus. The size of the nucleus is in the order of one hundred thousand times smaller than the size of the atom.

Furthermore, it was established that the nucleus of an atom consists of two types of particles, protons and neutrons. Protons have an electric charge which is equal, but opposite, to the electric charge of an electron. Neutrons do not have electric charge. Hence, for an atom to be electrically neutral, it has to have as many protons in the nucleus as there are electrons in its electron cloud. The number of protons in the nucleus of an atom determines its chemical properties. So, why does the nucleus need neutrons? The answer is that neutrons are needed to hold the nucleus together, since protons repel each other because of their electric charge. Protons and neutrons attract each other by a force, the nuclear force, which is nearly strong enough to avoid that protons are expelled. It needs just the right amount of neutrons to make a nucleus stable. Just a few more or less neutrons also do the job, giving rise to atomic isotopes with the same amount of protons but with a different amount of neutrons in the nucleus. Their chemical properties are the same, as it only depends on the number of electrons, hence the number of protons, but their atomic weights are different.

This picture of nature was complete by about 1930, light being electromagnetic waves, atoms and their chemical properties fully explained. But, then came the surprises. First, the theory of electrons also predicted the existence of the antiparticle of an electron, the positron. Second, an isolated neutron was discovered not to be stable but to disintegrate into an electron and a proton with an average lifetime of about 15 minutes. In itself that is not disturbing as long as neutrons in the atom are stable. But, in order to explain for neutron disintegration the existence of a new particle, the neutrino, had to be assumed, in order to account well for the energy balance and also for the balance of total angular momentum. Third, in order to explain the nuclear force, the existence was predicted of three new particles, the pions.

So, although it seemed unnecessary, nature had to contain more particles than photons (light particles), electrons, protons and neutrons. Indeed such particles were discovered. Positrons, pions and neutrinos were not only inventions of the human mind, but really existed in nature. Moreover, on performing the experiments for detecting those particles, yet other particles were discovered. Particles which are similar to the pions, but more heavy, the mesons, and other particles which are similar to protons and neutrons, but also more heavy, the baryons. Furthermore, the electron and positrons have like particles, muons and antimuons, taus and antitaus, whereas, moreover, the neutrinos come in three species, electron-neutrinos, muon-neutrinos and tau-neutrinos. It slowly became clear that, although maybe of not much relevance for the present state of matter, those particles may have had their part at the creation of the Universe. Hence, if we want to dominate the forces in our Universe, it seems urgently necessary that we study the properties of those particles and the associated forces, the strong and the weak nuclear forces. Much effort has gone into this branch of research in the second half of the twentieth century and is still going on.

Particle detection

Particles were detected by means of stacks of photographic plates in the early years of the twentieth century. Charged cosmic-ray particles which pass through such stack, leave spots on the plates. One may then reconstruct the track of the particle and estimate its mass. Later, ionization chambers were used, closed containers which are partly transparent in order to observe the interior and filled a supersaturated vapor which ionizes, leading to condensation droplets, when a charged particle passes through it. One observes trails of condensation droplets indicating the track followed by the charged particle.

The bubble chamber works on the same principle, but uses supersaturated hydrogen vapor. This is very dense in vapor particles and thus enhances the possibility of condensation formation. Moreover, liquid hydrogen can easily turn into supersaturated hydrogen vapor by controlling the pressure. That way one can erase the condensation trails by just increasing the pressure, thereby turning hydrogen vapor into liquid hydrogen. By subsequently lowering the pressure, the chamber is again ready for the passage of new charged particles. This technique is used to study the products of collisions between charged particles which are directed through the bubble chamber and protons. A bubble chamber full of liquid hydrogen is filled with protons, since the nucleus of a hydrogen atom consists of just one proton. Each charged particle which is created in the collision leaves a track in the chamber. By taking photographs one can lateron study those tracks.

Protons are moreover surrounded by a cloud of pions and other mesons. Consequently, charged particles which are directed through the bubble chamber, may also scatter with one of those particles. An artistic view of such process and the final bubble photograph is shown in the animation below.


Particles like pions, kaons, protons and some other mesons and baryons can leave tracks of several centimeters to meters. Hence, those tracks can be easily detected. However, most mesons and baryons live too short to be detected that way. They rapidly disintegrate into other particles which on their turn end up as protons, pions, kaons, electrons and positrons. The latter can be detected, whereas out of their tracks one can then reconstruct the mass and other properties of the original short-lived particle.

A disadvantage of the bubble chamber is that one can only take and lateron analyse a limited amount of photographs per year. Possible events which occur rarely would need thousands of years of detection with the bubble chamber. Nowadays, one uses several different techniques which involve a lot of fast electronics in order to allow for the analysis by computer of millions of events per second.

A popular experimental setup was the collision of electrons and positrons. A beam of electrons was directed headon to a beam of positrons, such that in the interaction point electrons and positron had equal but opposite velocities. From the interaction point emerged new particles, besides scattered electrons and positrons. On concentrating on the production of specific combinations of final particles, for example two oppositely charged pions, one could detect whether they emerged from something new. Thereto the total energy of the two beams was varied. At each energy one simply could measure the amount of times that the two pions of the example emerged. When, at a certain energy, the amount would be much more than at neighbouring energies, one concluded that a new thing had been formed which had decayed into the two pions. In a graph of amount of events versus the total energy of the beams, one observes such new phenomena as a kind of mountains, enhancements. The peak of the mountain indicates, via the famous formula of Einstein, the mass of the new particle, whereas the width of the mountain, measured at half hight, indicates, via the famous formula of Heisenberg, the lifetime of the particle, the larger the width, the smaller the lifetime. Some of those new particles live a very short fraction of a second. Many new mesons and baryons have been observed this way.

A huge step forward in understanding all those new subatomic particles was the assumption that they themselves consist of a new type of particles, which were baptised quarks and antiquarks. Baryons were analysed to consist of three quarks, whereas mesons had to be formed of one quark and one antiquark. The so-called quark model has been studied over the past fifty years by many scientists. Thousands of experiments have been performed at special laboratories with powerful particle accelerators. Me and my collaborators have concentrated on the study of mesons.

Newly found enhancements in the experimental results were often reported to the press. But with more and more new enhancements at hand, it became increasingly more difficult to find agreement with all kind of different quark models. Hence, the wildest speculations came up in order to explain the newly found enhancements. However, besides me and my present collaborator, nobody seems to have observed that there exist four types of enhancements in observations related to strong interactions. Only one of those four types can be associated to higher mass states of quark configurations. The other three are either accidental or the consequence of the phenomenon that quarks and antiquarks can be created pairwise out of energetic matter.


In the video I show an animation for the process of the annihilation of an electron with its antiparticle, the positron. What remains after their annihilation is a lump of energetic matter. When such object has enough energy it allows for the simultaneous creation of a quark and an antiquark. In the video I take as an example the creation of a rather heavy beauty quark and its antiparticle, an equally heavy beauty antiquark, out of what results from electron-positron annihilation. That system is a structure which eventually could form a meson. But only if it has the right amount of energy. If that is the case the beauty quark and the beauty antiquark start resonating, a process which makes them stay together a bit longer instant of time. The closer their energy approaches the right value for one of the beauty-antibeauty meson modes, the better they resonate. Eventually that system desintegrates into lighter particles but the amount of lighter particles which comes out of the region where the electron and the positron have annihilated is much larger than for energies far away from the right value.

Such process is like a kind of an open door. When the electron and the positron sense that the door is completely open for the formation of beauty-antibeauty meson modes they more likely annihilate and pass the door which is open for them in order to to convert into other types of particles. When their energy is distant from the right one, they find the door almost closed.

Knowledge of the masses of all possible beauty-antibeauty meson modes teaches us the details of the strong interactions, similar to atomic masses and their excitations telling us about the electromagnetic interactions. Hence, involving enhancements which do not classify as beauty-antibeauty meson modes gives a distorted picture of their real spectrum and, as a consequence, the wrong conclusions for strong interactions.

We discovered a very important property for the resonance modes for mesons. Namely, all systems which consist of one quark of any type and one antiquark of a different or the same type resonate with the same oscillation frequency. As a result the difference in mass of any two subsequent possible masses for meson modes is constant and the same for any quark-antiquark combination. This result is in conflict with the result of the most popular model for mesons, lattice QCD and the funnel potential which is derived from it, which predicts that for growing masses the mass difference decreases and is not equal for all quark-antiquark combinations. However, it is not in conflict with experiment. On the contrary, experiment strongly suggest our observation.

The above observation can only be reproduced by a model which describes all steps of the experimental conditions, from the situation of the initial particles before annihilation to the state of the end products after disintegration. This procedure has moreover a second important message. Namely, the creation of the light quark-antiquark pair which is responsible for the disintegration of the system is related to a quantum with a surprisingly light mass.

It could have been that the latter quantum only could exist in the interior of a meson which would have made its detection extremely difficult. In the video I describe that it is observed to also exist as a free particle, the higgs boson for strong interactions.

What is the evidence?

So in all I have made a series of observations which all point towards the existence of the higgs boson for strong interactions. Below I will summarise them.

1. Micro-Universes

In a theoretical model for confinement it is described how hadrons can be considered as micro-Universes. Very much like our Universe which is governed by gravity, the hadronic micro-Universes are formed by the gluon interactions. As such quarks and gluons are confined to a finite region in space. Now, in principle, such Universes are infinitely large because its habitants, quarks and gluons, do not have knowledge of anything outside their Universe. This would be true if they could only interact amongst each other. But quarks are also electrically charged and may thus interact with photons. Consequently, a more complete description of hadrons needs the inclusion of electromagnetic interactions. But, photons do not interact with gluons. So, they are not confined to hadrons but can freely move through the interior and the exterior of the hadronic micro-Universe. This then leads to a model in which the particles obey to two different worlds. One is the world of the photons, the other the world of quarks and gluons. Were it not for the electrical charge of the quarks, they would not even have knowledge of each other, because photons would pass through an hadronic micro-Universe without noticing its presence, whereas the quarks and the gluons would not observe the photon which passes through their micro-Universe. The complete construction of such overlapping worlds is based on the assumption of the existence of two Higgs fields. One of those Higgs fields must be associated to a light Higgs particle, the higgs boson for strong interactions.

2. The universal frequency of 190 MeV

Another important feature of the hadronic micro-Universes is that everything inside them moves with the same rythme, leading to the equidistant spectra of mesons. However, in order to compare that finding to the experimental observations one must then also include the consequences of quark-pair creation which eventually may lead to the process of disintegration of one hadronic micro-Universe into two or more hadronic micro-Universes. Me and my collaborators have set out on that task in the late seventies. Step by step we have shown that such an approach describes very well the results of the various experiments in particle physics. It is actually at present the only model which goes beyond bump hunting and describes the full structure of the experimental cross sections. Many bump-hunting models have been developed in the past three decades. All with one success or another, meaning that with some perturbative methods it was managed to agree with the masses of one or the other enhancement, but not with the very complex meson spectrum as a whole.
Moreover, experimental results have been very disappointing in the past three decades. Large parts of the higher excitations of the meson spectrum have not been extensively searched for, whereas also many fine details of the lower part of the mesonic spectrum are not further studied. So, we are actually dealing with a very cripple insight in the structure of the mesonic spectrum.

These are 2009 data for charmonium vector mesons, composed of a charm quark, a charm antiquark and glue. The resonances observed below 4.5 GeV were known for more than three decades. The signal above 4.5 GeV is not enough to observe clearly further resonances.

Nevertheless, whatever new enhancements showed up in experiment, we have not only found them in agreement with our model predictions, but we have, based on our model predictions, pointed out the existence of enhancements in the experimental data which had not been observed by our experimental colleagues.
However, in order to observe the equidistance in the meson spectrum, one needs the discovery of lots of higher excitations. From the present experimental situation, it is difficult to find a sufficient number of higher excitations of quark-antiquark mesons, in order to establish their mass differences. For many quantum numbers only the ground state, or just one more excited state has been observed in experiment.

light-quark mesons

In particular for the light quarks, up, down and strange, almost no data are available to verify the mass separations. The only system which has some excited states are the so-called tensor states. The available data are shown in the table below (1 GeV = 1000 MeV). More detailed information on that system, in particular on the flavor assignments and the reason why the ground states are not included in my analysis, can be found in the following conference proceedings.




In those data, one observes indeed a perfect agreement with our model predictions, namely that the spacings between the masses of excited states should be of the order of some 380 MeV which is exactly two times the frequency as expected. Small deviations depend on the precise environment of disintegration channels for each state separately and on the accuracy of experiment.

charm-strange mesons

For the quark-antiquark system with one charmed and one strange quark, I have observed enhancements which could possibly show that for that system our model predictions are in good agreement with experiment. In the table below one can see how our model interprets the data. The first column refers to our assignments for the various states. The blue states are those which are found by experiment, or by me in experimental data. Given that the masses of pure micro-Universe confinement are the same for 2S and 1D states, for 3S and 2D states etc., I have put them on the same horizontal line in the table. Our full calculation for the mesonic cross section results for the D states in enhancements at masses which are some 10-50 MeV different from the masses given by pure micro-Universe confinement, whereas for the S states the enhancements come some 150-200 MeV below the masses which follow from pure micro-Universe confinement. Only the 1S state, which has no D state partner, deviates usually more than 250 MeV from the mass than predicted by pure micro-Universe confinement. The latter predictions are given in the second column, under HO mass of the table below. HO stands for harmonic oscillator, which gives coincidentally the same spectrum as pure micro-Universe confinement.
The terms S states and D states stem from the ideas of confinement models. However, the enhancements which are observed in experiment are mixtures of the two types of resonances. Hence, in analysing quark distributions of those states, one might come to the conclusion that what we here call a D state should be an S state since even a small fraction of S state could dominate the properties of the mixture.
In the third column I have collected the observations which I will discuss below.

List of vector charm-antistrange resonances:
state    
HO mass (MeV)
observations
   
1S
2355
D*s (2112)
2S, 1D
2735
2S and 1D   D*s (2710)
3S, 2D
3115
3S and 2D
4S, 3D
3495
4S and 3D
5S, 4D
3875
 
6S, 5D
4255
  Z+(4050) and Z+(4250)  
7S, 6D
4635
Z+(4430)


Inclusion of meson loops results in mass shifts,
some 150-200 MeV for S states and 10-50 MeV for D states.

MZ+(4430) - MZ+(4050)  = 380 MeV.


  


Below we present the experimental results obtained by the BABAR collaboration.



The 1S is well established, hence does not have to be discussed here. However, the 2S state should give an enhancement very close and just above the well-established Ds1(2536) state which does not belong to the set of states we discuss here. The latter gives a very pronounced peak of about 10000 events, whereas the 2S state of the set of states under consideration seems to be the little peak at about 2.57 GeV which contains about 1500 events (upper left figure). Now, in order to see this better, BABAR should diminish the data separation in that mass area, which with 1500 events per 20 MeV could well be done and still give 150 per 2 MeV for example.
At present the peak at 2.71 GeV (upper middle figure) is interpreted as the 2S state, whereas, according to our model, that should be the 1D state. Let me repeat here that the terms S states and D states stem from the ideas of confinement models. However, the enhancements which are observed in experiment are mixtures of the two types of resonances. Hence, in analysing quark distributions of those states, one might come to the conclusion that what we here call a D state should be an S state since even a small fraction of S state could dominate the properties of the mixture.
Further enhancements in the data are not analysed so far by any experimental collaboration. I give here in the above figure our interpretation. The Ds(23P0,2) state does not belong to the set of states under consideration.
Our interpretation also allows to include the so-called Z+ states which were discovered by the Belle collaboration, but not yet confirmed by other collaborations.

The above observations are published in conference proceedings.

charm-charm mesons

For charm-anticharm mesons, I found indications in experimental results for the existence of several more charmonium mesonic vector states (mesons consisting of one charmed quark and one charmed antiquark).
First there are the ψ(3D), ψ(5S) and ψ(4D) which were visible in the cross sections published by the Belle collaboration, exactly where our full cross-section model predicts them.



Then I found the same states in the missing signal of π+π- J/ψ production published by the BABAR.



Particularly nice signals for the ψ(5S) and ψ(4D) which were visible in the ΛcΛc cross sections published by the Belle collaboration.



Further indications for those excitations of the charm-anticharm system I found in other cross sections published by the Belle collaboration.

  
   
   


Neither Belle, nor BABAR performed a more detailed inspection of those states. Our work on this issue was not accepted for publication.
I also found signals for the more higher charmonium excitations ψ(6S) and ψ(5D) which were visible in the missing signal of π+π- J/ψ production published by the BABAR collaboration.



BABAR did not perform a more detailed inspection of those states.
Finally, in the charmonium spectrum, I also found signs for the even higher excitations ψ(7S), ψ(6D) and ψ(8S) which were visible in the D*D* of cross sections published by the BABAR collaboration. The blue crosses (X) at the axis show where we predict the D states. Those states one can predict from our model without much effort within some 10-50 MeV accuracy. Their precise masses and shapes of the cross sections and those of the S states need a full model calculation. Nevertheless, it is save to say that nS states come some 150±50 MeV below the corresponding (n-1)D states. No D state corresponds to the 1S state. The masses of the 1S states come out, by a full model calculation, usually much lower, some 300±50 MeV, than is predicted by pure micro-Universe confinement.



Our results were published in the Chinese Physics Journal. BABAR did not perform a more detailed inspection of those states.

A next succes was obtained in the upsilon (beauty-antibeauty) spectrum where I found a clear signal of the Υ(2D) in data published by the BABAR collaboration exactly at the mass which is predicted by our model.



BABAR did not perform a more detailed inspection of those states. Our work on this issue was not accepted for publication.

Several misconceptions, which still run in the particle physics community, have to be addressed.

Mesonic spectra are equidistant

That issue has been dealt with in the above, the equidistant spacing of the masses of higher excitations as predicted by our model for confinement which considers hadrons as micro-Universes. In the figure below you may compare our predictions with the observed spectrum and the predictions of lattice QCD.



The yellow lines are the predictions from pure micro-Universe confinement. Our cross-section model turns this in the red, observed, spectrum. The black lines are the predictions from the funnel potential which is derived from lattice QCD. No further comment.

Higher mesonic excitations have lifetimes comparable to,
or even smaller than, lower mesonic excitations

Another misconception is that the widths of resonances become larger and larger when you go higher up in the spectrum of states.
That issue has been dealt with in 1983 by me in a work which is dedicated to the amount of possible configurations which are possible in meson disintegration. I found then that the number of possible configurations grows exponentially when you go higher up in the spectrum. This means, for example, that a ψ(5D) state at disintegration has many more possible configurations than a ψ(1D) state. Now, many of those possible configurations do not contribute to disintegration because the ψ(5D) has not sufficient mass to allow their production. The few configurations which do contribute to disintegration have, however, a relative small probability to occur, because they must compete with all the other possible configurations. As a consequence the ψ(5D) may exist a longer time (has a larger lifetime) since it has to wait untill a configuration occurs which allows for disintegration. The ψ(1D), however, has much less possible configurations for disintegration. The few of those which by their masses allow for disintegration occur much more often because they do not have to compete with so many other configurations. Hence, the ψ(1D) lives shorter then the ψ(5D), which reflects itself in a larger width in the cross section for the ψ(1D) than for the ψ(5D).
Of course, there are also other factors which determine the width in the cross section for a certain state. But, it is clear why hadronic widths do not grow for higher excitations.

There are less scalar meson states discovered in experiment
than there are predicted by our quark model

A further misconception is that there are many more states in the scalar meson sector than can be predicted by quark models and hence other fancy configurations of more quarks would be necessary to explain the experimental results in that sector.
That issue had been dealt with in a work which is dedicated to the scalar mesons. However, in that work we had not clearly stated what the model predictions were for the higher excitations. At that time, early 1980's, we found it already quite something that the unexplainable light scalar mesons just appeared without further effort in our cross-section model. Recently, I have remedied that omission with a more complete spectrum of scalar mesons. It shows that actually our model predicts more than what has been found yet in experiment.
Below, I give a table of our predictions for the up-strange scalar mesons, actually figures with cross sections and compared to data, would be better.
Instead of the central mass and resonance width, I present the real and imaginary parts of a complex quantity which determines whether an enhancement represents an up-strange scalar meson. The central mass of the enhancement is not far away from the real part of that quantity, whereas the resonance width equals about twice its imaginary part.
The masses are in GeV (1 GeV = 1000 MeV).

List of light scalar meson resonances:
 Real part (GeV) 
 2 × Imaginary part (GeV) 
 experimental state 
   
0.772
0.562
K0*(800)
1.52
0.194
K0*(1430)
1.79
0.104
 
2.04
0.30
K0*(1950)
2.14
0.13
 


The table continues forever,
but at a certain point
the enhancements become too feeble to be observed.




Now, each up-strange scalar meson makes part of a nonet of scalar mesons, which are grouped in four classes, two isosinglets, one isotriplet and the strange scalar mesons, which consists of two isodoublets. Scalar mesons within one class have the same, or at least very similar masses. The up-strange scalar meson is one of the four possible strange scalar mesons. Usually, one refers to a class, not to one of the scalar mesons of that class in particular.
Hence, associated with each of the strange scalar mesons of the above table, our model predicts three more classes of particles with masses which are somewhere not too far from the masses in the table. Several of those have not been discovered yet. Consequently, there is no need for fancy configurations.

Not every enhancement is a resonance

A further misconception is that each bump in the cross section is associated to a mesonic resonance.
In an extensive work we have discussed the issue of threshold enhancements in production experiments which have no relation to the mesonic spectrum. Later, we discussed several examples.
Below, we show one notorious example, the X(4640). It shows up as a large enhancement in the cross sections for ΛcΛc production, just above the ΛcΛc production threshold (lefthand side figure). Also visible are the higher charmonium excitations ψ(5S) and ψ(4D).
In the righthand side figure we show the cross sections for D*D* production. One notices that the ΛcΛc enhancement is considerably smaller in the D*D* cross sections.

  


The explanation is as follows. The Λc is a baryon which consist out of three quarks, one of which is charmed. Hence, to obtain a Λc and a antiΛc starting from a charm-anticharm meson, nature must create two pairs of quark-antiquark. Such processes occur much less frequently than the creation of one such pair. The reverse process is equally rare. Hence, to find ψ(5S) and ψ(4D) in the signal of pairs of Λc and a antiΛc is not very probable. The same argument holds for any other possible resonance. The large peak does not stem from a resonance, but is just an effect of the opening of the threshold for the creation of a Λc and a antiΛc.
In the direct channel D*D* it needs one pair of quark-antiquark to be created for the production of a Λc and a antiΛc, but also for the production of ψ(5S) and ψ(4D). Here, we find then accordingly a much smaller signal for threshold enhancement in ΛcΛc production.

Another work is devoted to the X(4260) enhancement. This already-baptised particle is found in a disintegration channel which does not make part of the configurations which I described above. It is so to speak a sneaky possibility of disintegration which occurs with much less frequency, but is indeed not impossible. Such channels have been observed before. In their enthousiasm over this new enhancement in the cross section of this channel, our colleagues overlooked an important phenomenon which is also visible in this cross section, namely the absence of enhancements for the already well-known mesonic resonances in this channel. This is even more dramatic in the case of the ψ(4S) where instead of an enhancement one observes a dip in the data. In the figure below I show with a blue line what should have been found at the mass of the ψ(4S). Experiment finds a dip.



Now, everybody may have experienced this phenomenon while he was taking a shower and someone decided to fully open the hot-water tap in the kitchen. Suddenly the water jet of the shower turned into small sprinkling device with, moreover, only cold water. Actually one can then measure the flow in the kitchen by the absence of it under the shower.
That is what I did with the data. I took the X(4260) signal, turned it upside-down and compared it with conventional data. The result is shown below.



The black squares are from the upside-down X(4260) signal, whereas the red dots are the conventional data. We find an excellent agreement.
We may thus conclude that the X(4260) signal is what has been left over from strong disintegration and that its structure must first be turned upside down before it should be analysed.

3. The mass associted to quark-pair creation

We have discussed above that the main mode of the disintegration of a quark-antiquark meson is through quark-pair creation. This mode is the basic ingredient of our cross-section model with which we reproduce the experimental cross secions. I had found that the intensity of that process is associated to a quantum of about 30-40 MeV in the early 1980's. However, at that time there was no quantum discovered which could minimally be related to a mass of 30-40 MeV. So, I had to let my observation rest for a while. But, I never forgot the issue. Hence, when data came up which could relate to such mass, I did get on high alert and started to sift through many experimental results. Below, I give an account of what I discovered.

4. Oscillations in cross sections for mesonic resonances

While studying the X(4260) signal, I found the first indication for the existence of the E(38), the higgs bosons of strong interactions,



namely a tiny oscillation in the cross section of the X(4260).
After that result, I sifted through many data from a variety of experiments. But, as one may observe from the above figure, it is necessary that the distances between data points are substantially smaller than the 76 MeV of the oscillation period. Unfortunately, most experimental results come with larger separations between the data points, or are full of resonances and other structures which give too much unrelated variations in the signal. Not much data could show to some degree of satisfaction what I was looking for. In the end I came up with only three more examples.



In the above figure I show the oscillations in experimental cross sections, or in event distributions, for three different types of quark configurations. In the upper figure the experimental data were obtained for up-antiup (mixed with also down-antidown) mesonic systems. The middle figure contains data for charm-anticharm, whereas the lower figure refers to beauty-antibeauty. Three very different systems which show the same oscillation frequency of about 76 MeV. This underlines, once more, that mesonic systems oscillate with the same rythme, whether they consist of light quarks, up and down, heavier quarks, charm, or very heavy quarks, beauty.

None of the experimental collaborations have further inspected this phenomenon. The three additional pieces of evidence were not accepted for publication. According to me the oscillations occur because of the interference between the E(38) and the frequency of 190 MeV with which the quarks oscillate inside the hadron.

5. The hybrid of the Υ(2S)

While studying the before mentioned experimental results, where I discovered a clear signal of the Υ(2D) and a feeble signal of the Υ(1D) in data published by the BABAR collaboration exactly at the masses which are predicted by our model, I was also searching for one more piece of evidence for the oscillations. However, what I then discovered was far more interesting, namely the first direct indication of the existence of the E(38).



At just a little less than 38 MeV (indicated by the vertical line) I found the first ever hybrid, which is a configuration of a normal quark-antiquark hadron, the Υ(2S) in this case, and which contains also an excitation of the glue, the E(38).
This is a very exciting result since it proofs that the E(38) does exist. But, for now, we only have shown its existence in the interior of the hadron:
BABAR did not perform a more detailed inspection of this state. The additional piece of evidence was not accepted for publication.

6. Direct indications for the E(38)

The most exciting result came however when, with the same data, I studied the cross sections for the transitions Υ(2S)→Υ(1S). The black histogram in the figure below corresponds to the data obtained by the BABAR collaboration, whereas the green histogram represents a normal distribution as expected for the experimental spreading of masses. In red, at the bottom of the figure, I have indicated the difference between the black and the green histograms.
The green histogram is chosen such that the amount of difference (the number of events) is equal on both sides of the central Υ(1S) mass which is indicated by the vertical black line. Hence, the total missing signal above the Υ(1S) mass is equal to the total excess of signal below the Υ(1S) mass.
In itself it is not surprising that there is some asymmetry in the signal for the process Υ(2S)→Υ(1S). That can be explained by so-called Bremsstrahlung, although in the specific case under consideration it is supposed to have a rather small effect. But, what is exciting, is that I observed some feeble structure in the asymmetry.



One observes a perfect dip in the cross section, right 38 MeV above the central mass of the Υ(1S) and moreover a corresponding enhancement which sets out from 38 MeV below the central mass towards lower masses.
The latter signal can be explained by the production of an unaccounted for E(38) since that mass or more (the E(38) will also take some kinetic energy) will then be missing. As a consequence extra events will be found with masses which are 38 MeV or more below the central Υ(1S) mass. We observe, moreover, similar peaks which set out towards lower masses at about twice and at about three times the E(38) mass below the Υ(1S) mass.
The dips for masses above the Υ(1S) mass come at about the same masses and can be explained by the disintegration of the hybrid for the Υ(2S) we had found.

Now, since the observed structure is feeble, as I mentioned before, it could just be due to statistical fluctuations, imperfections in the experimental results which would only cancel out if one had an infinity of data. So, I needed some kind of confirmation, which indeed I found.

In the figures below I show different processes, namely the transitions Υ(3S)→Υ(1S) and Υ(3S)→Υ(2S). Unfortunately, there are much less events observed for those transitions by the BABAR collaboration. As a consequence I had to select larger separations for the data points, which obscures a little bit the observation of the E(38). For the transition Υ(2S)→Υ(1S) I could select separations of 9 MeV, but for the transition Υ(3S)→Υ(1S) a had to select 19 MeV separations, whereas the amount of data for the transition Υ(3S)→Υ(2S) only allowed for separations of 38 MeV.



In the above figure I show the difference signal for the transition Υ(3S)→Υ(1S). One observes the dips at 38 MeV and at 76 MeV above the Υ(1S) mass. The dip at 114 MeV above the Υ(1S) mass is absent. Furthermore, one observes excess at all three masses below the Υ(1S) mass. However, the signal at 114 MeV is rather small.



In the above figure I show the difference signal for the transition Υ(3S)→Υ(2S). One observes the dips at 38 MeV, at 76 MeV and at 114 MeV above the Υ(1S) mass. Furthermore, one observes excess at 38 MeV and at 76 MeV below the Υ(1S) mass. The exces at 114 MeV below the Υ(1S) mass is absent.

So, more confirmation would be in place. This I could achieve from yet a different process, but then I had to consider all possible transitions together in order to have sufficient data. Also, in this process the Bremsstrahlung asymmetry cannot be ignored. Nevertheless, some structure, indicated in green, could be observed.



Notice that indeed the Bremsstrahlung asymmetry is here very well observable, namely the broad enhancement for masses below the Υ(1S) mass, whereas it was basically absent in the previous processes.

With this final result I was already more confident that I had enough evidence for the existence of the E(38). Nevertheless, I started looking for a publication of the BABAR collaboration where maybe they had done a similar analysis. Indeed, I found such publication where a study had been performed which is similar to mine. The result of this study, for a larger set of data than I had at my disposal, is shown below.



I have indicated the dips and excesses in red, the reader may conclude for himself.

BABAR did not perform a more detailed inspection on the existence of the E(38). The additional piece of evidence was not accepted for publication.

Let us consider the evidence so far for the existence of the E(38) and its two replicas.